Question

What volume and mass of steam at 100 Celsius and 760. torr (or 1 atm) would release the same amount of energy as heat during condensation as 65.5cm^3 of liquid water would release during freezing?

Answer 1

To release the same amount of energy during condensation as 65.5 cm³ of water does during freezing, 0.538 moles or 9.68 g of steam is required, which is equivalent to a volume of approximately 12.05 L at 100°C and 1 atm.

Step-by-step explanation:

To determine the volume and mass of steam at 100 Celsius and 760 torr that would release the same amount of energy as heat during condensation as 65.5 cm³ of liquid water would release during freezing, we need to consider the heat of condensation and the heat of fusion for water. Since 1 mole of water vapor at 100°C condenses to liquid water at the same temperature releasing 40.7 kJ of heat, the amount of energy released during the freezing of 65.5 cm³ of liquid water is equivalent to the amount of energy required to melt the same volume of ice.

Knowing that the density of water is approximately 1 g/cm³, we can calculate the mass of the 65.5 cm³ of water as 65.5 g, which is close to 65.5/18 ≈ 3.64 moles of water (since the molar mass of water is 18.0 g/mol). The heat of fusion for water is roughly 6.01 kJ/mol, so the energy released during the freezing of 65.5 g of water is 3.64 moles × 6.01 kJ/mol = 21.9 kJ.

To release the same amount of energy as 21.9 kJ during condensation, we would need 21.9 kJ / 40.7 kJ/mol ≈ 0.538 moles of steam. Since the molar volume of a gas at standard temperature and pressure (STP) is 22.4 L/mol, the volume of steam required would be 0.538 moles × 22.4 L/mol ≈ 12.05 L of steam. The mass of this steam would be 0.538 moles × 18.0 g/mol ≈ 9.68 g.

Answer 2

Step-by-step explanation:

The given data is as follows.

Volume = 65.5
`cm^(3)`

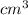
Temperature =
`100^(o)C`

Pressure = 760 torr or 1 atm

As we know that q =
`mL_(f)`

where, m = mass

`L_(f)` = latent heat of fusion

As density of water is 1.0 g/ml. Hence, mass of water in liquid state is calculated as follows.

mass = density × volume

=
`1.00 g/ml * 65.5 ml` (as 1 ml = 1
`cm^(3)`)

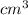= 65.5 g

As heat of fusion of ice is 333.55 J/g. Hence, calculate the heat energy as follows.

q =
`mL_(f)`

=
`65.5 g * 333.55 J/g`

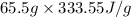
= 21847.52 J

And, heat of vaporization of water is 2257 J/g.

Also, q =
`mL_(v)`

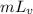
where,
`L_(v)` = heat of vaporization

Therefore, we will calculate the mass of steam as follows.

q =
`mL_(v)`

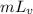
21847.52 J =
`m * 2257 J/g`

m = 9.68 g

It is known that density of steam water is 0.0006 g/ml. Hence, calculate the volume of steam as follows.

Volume =
`(mass)/(Density)`

=
`(9.68 g)/(0.0006 g/ml)`

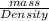
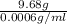
= 16133.33 ml

or, = 16.13 L (as 1 L = 1000 ml)

Thus, we can conclude that 9.68 g of steam condenses will release the same amount of energy as 65.5 g of freezing liquid water and its volume is 16.13 L.