Question

Given the equation Square root of 8x plus 1 = 5, solve for x and identify if it is an extraneous solution.

A)x = One fourth, solution is not extraneous
B)x = One fourth, solution is extraneous
C)x = 3, solution is not extraneous
D)x = 3, solution is extraneous

Answer 1

an extraneous solution is a root that when substituted to the original equation, the answer becomes invalid. In this respect, the equation is square root (8x + 1) = 5; 8x + 1 = 25; x1 = 3; 8x + 1 = -25 ; x2 = -26/8 = -13 /4. Upon substitution, square root of (8*3 + 1 ) = 5, this is valid. Hence the answer to this question is C.

Answer 2

For this case we have the following equation:

`√(8x+1)=5`

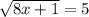
From here, we must clear the value of x.

For this, we follow the following steps:

1) We raise both members of the equation to the square:

`8x+1=25`

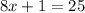
2) We pass the number 1 subtracting:

`8x = 25 - 1  8x = 24`

3) We pass number 8 dividing :

`x =(24)/(8) = 3`

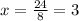
We observe that the given equation can be evaluated for the solution obtained.

Therefore, the solution is valid.

Answer:

C) x = 3, solution is not extraneous