Given
Velocity of boat relative to water,

Direction of current towards east,
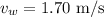
To find
What is the boat's speed relative to the ground when it is heading east, with the current, and west, against the current?
Step-by-step explanation
When the boat moves along the current,
The velocity of the boat is

When the boat travels opposite to the current direction
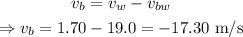
Conclusion
The velocity of boat along the current is 20.70 m/s.
The velocity of boat against the current is -17.30 m/s.