Question

If f(x) =tan(2x) then f'(pi/6)?

Answer 1

The derivative of f(x) = tan(2x) is
`2sec^2(2x)`. When
`x = \pi /6,` the derivative is 8/3.

Step-by-step explanation:

To find the derivative of f(x) = tan(2x), we can use the chain rule.

The derivative of tan(x) is
`sec^2(x)`, so the derivative of tan(2x) is
`2sec^2(2x).`

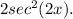
Substituting π/6 for x, we have -

`f'(\pi /6) = 2sec^2(2(\pi /6))`

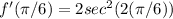
= 2sec^2(π/3).

Using the identity
`sec^2(\pi /3) = 4/3,`

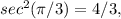
we find that
`f'(\pi /6)` = 2(4/3)

= 8/3.

Answer 2

You should try an online calculator called tiger algebra...it really good and can answer question like these ones