Answer:
16.94% probability that he will pass
Explanation:
For each question there are only two possible outcomes. Either the answer it correctly, or he does not. The probability of answering a question correctly is independent of other questions. So we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.
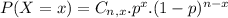
In which
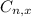 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.
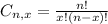
And p is the probability of X happening.
6 questions
So n = 6.
4 possible answers
So
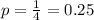
He passes if he gets atleast 3 questions right.what is the probability that he will pass
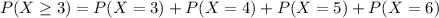
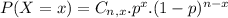
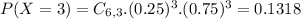
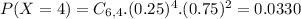
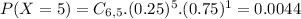
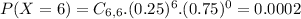

16.94% probability that he will pass