Question

Graph the function f(x) = x4 − 7x3 + 12x2 + 4x − 12 using graphing technology and identify for which values of x the graph is increasing.

Answer 1

Value of x is increasing between
`-0.147<x<2` and
`x>3.397`

Explanation:

Given : Function
`f(x)=x^4-7x^3+12x^2+4x-12`

To find : For which values of x the graph is increasing.

Solution :

We have plot the graph of the given function
`f(x)=x^4-7x^3+12x^2+4x-12`

The graph is attached below.

We see that the value of x is increasing from the interval

A-
`(-0.147,-12.306)` to
`(2,4)`

B-
`(3.397)` to
`(\infty)`

Which means x value is increasing between

`-0.147<x<2` and
`x>3.397`

Answer 2

we have

`f(x) = x^(4) - 7x^(3) + 12x^(2) + 4x - 12`

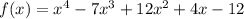
The options of the question are

A. X:-3 to x:-2

B.x:-2 to x:-1

C. X:1 to x:2

D. X:2 to x:3

Using a graph tool

see the attached figure

The graph is increasing in the interval (-0.15,2) and the interval (3.4, ∞)

The graph is decreasing in the interval (-∞, -0.15) and the interval (2,3.4)

therefore

the interval (1,2) is included in the interval (-0.15,2)

the answer is the option

C. X:1 to x:2