Answer:
The coordinates of point R are
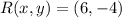 .
.
Explanation:
From Geometry we understand that magnitude of Diameter is twice the magnitude of Radius. Vectorially speaking, both radius and diameter are collinear. That is:
 (1)
(1)
Where:
 - Vector radius.
- Vector radius.
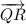 - Vector diameter.
- Vector diameter.
If we know that
 and
and
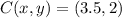 , then coordinates of point R are, respectively:
, then coordinates of point R are, respectively:
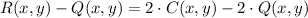
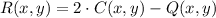
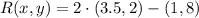
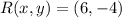
The coordinates of point R are
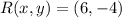 .
.