Question

Find the equation of a line (in y=mx+b form) through the point (-2,5) with an angle of inclination of 45°

Answer 1

Ok, so

Let me draw the situation here below:

Remember that the slope of a line is given by the formula:

`\begin{gathered} m=\tan (\theta) \\ m=\tan (45)=1 \end{gathered}`

The slope of our line will be 1.

Now, we could use the equation:

`y=y_1+m(x-x_1)`

Where (x1,y1) is a point of the line and m is the slope. Replacing our values:

`\begin{gathered} y=5+1(x-(-2)) \\ y=5+x+2 \\ y=x+7 \end{gathered}`

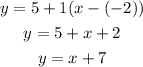
Therefore, the equation is y=x+7.