Question

For what numbers theta is f(theta)=tan(theta) not defined? f(theta)=tan(theta) is not defined for numbers that are (even multiples, odd multiples, multiples) of (45*, 90*, 180*). Select an answer from each set of parentheses.

Answer 1

Hence,
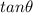`tan\theta` is not defined for odd multiples of
`90^(\circ)`.

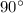Explanation:

We are given that
`f(\theta)=tan(\theta)`

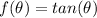
We have to find the value of theta for which
`tan\theta`

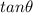
We know that when
`sin90^(\circ)=1`

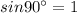
and
`cos90^(\circ)=0`

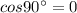
Then
`tan90^(\circ)=(sin90^(\circ))/(cos90^(\circ))`

Substituting values

`tan90^(\circ)=(1)/(0)`

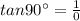
`tan90^(\circ)` is not defined .

If we take

`\theta=45^(\circ)`

`tan45^(\circ)=(1)/(\sqrt3)`

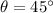
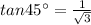
Hence,
`\tan\theta`is defined for odd multiples of
`45^(\circ)`.

If we take
`\theta= 180^(\circ)`

Then
`tan180^(\circ)=(sin180^(\circ))/(cos180^(\circ))`

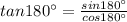
We know that
`sin180^(\circ)=0\;and cos90^(\circ)=-1`

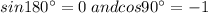
Therefore.
`tan180^(\circ)=(0)/(-1)=0`

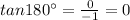
We know that 180 is even multiple of 90 and even multiple of 45 .Hence, we can say
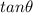`tan\theta` is no defined for odd multiples of 90

because
`tan(3\pi)/(2)=tan(2\pi-(\pi)/(2))`

`=-tan(\pi)/(2)`

`=-tan90^(\circ)`

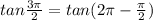
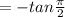
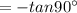
=not defined.

Hence,
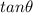`tan\theta` is not defined for odd multiples of
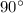`90^(\circ)`.

Answer 2

The numbers of theta that results to an undefined number for tan theta is odd multiples of pi/2. This means the answer to this problem is odd multiples of 90 degrees. you can try a calculator to find the answer since tan 90 is equal to 1/0 equal to infinity which is then undefined