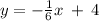Question

What is the equation of the line in slope-intercept form?

Answer 1

The equation of the line in slope-intercept form is:

`\:y=-(1)/(6)x\:+\:4`

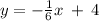
Explanation:

The slope-intercept form of the line equation

`y = mx+b`

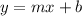
where

• m is the slope

• b is the y-intercept

Given the points on the line graph

• (0, 4)

• (3, 3.5)

Determining the slope between (0, 4) and (3, 3.5)

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`m=(3.5-4)/(3-0)`

`m=((7)/(2)-4)/(3-0)`

`m=-(1)/(6)`

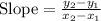
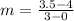
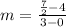
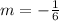
Thus, the slope of the line = m = -1/6

We know that the value of the y-intercept can be determined by setting x = 0 and determining the corresponding value of y.

From the graph, it is clear

at x = 0, y = 4

Thus, the y-intercept b = 4

now substituting b = 4 and m = -1/6 in the slope-intercept form

`y = mx + b`

`\:y=-(1)/(6)x\:+\:4`

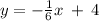
Therefore, the equation of the line in slope-intercept form is:

`\:y=-(1)/(6)x\:+\:4`