Question

A sine function has a maximum y value of 8 and a minimum y value of -2. The period of the sine function is 4pi, and there is no phase shift/horizontal translation. What is the equation of the sine function?

Answer 1

`\displaystyle y = 5\sin((1)/(2) x) +3`

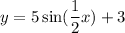
Explanation:

The Sine Function

The general form of the sine function (with no phase shift) is:

`y = A\sin(\omega x) +M`

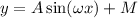
Where:

A = Amplitude

ω = angular frequency

M= Midline or vertical shift

The midline can be calculated as the mean value of the maximum and minimum values of the oscillation, thus:

`M=(8-2)/(2)=3`

The amplitude is half the difference between the maximum and minimum values of the oscillation:

`A=(8+2)/(2)=5`

The angular frequency is calculated in terms of the period T as:

`\displaystyle \omega=(2\pi)/(T)`

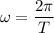
Since T=4π:

`\displaystyle \omega=(2\pi)/(4 \pi)=(1)/(2)`

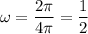
Substituting in the general form of the sine function:

`\boxed{\displaystyle y = 5\sin((1)/(2) x) +3}`

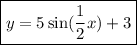
The first choice is correct