Answer:
- The explicit equation is given by:
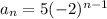
- The domain of the geometric sequence is: All the natural numbers (i.e. n≥1)
Explanation:
Explicit formula--
The explicit formula is a formula which is used to represent the nth term of a sequence in terms of the variable n.
It is given that:
The first term of the sequence is 5 and the second term is -10.
This means that if a denotes the first term and r denotes the common ratio.
The geometric sequence is given by: a,ar,ar²,ar³,....
i.e. the nth term of the sequence is given by:
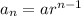
Then we have:
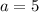
and
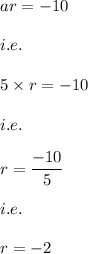
Hence, the nth term of the sequence is given by:
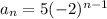
We know that the domain of a geometric sequence is the set of all the natural numbers.
( since the term a_n is defined for all the natural numbers ).