Question

William has sold 95, 41, 84 and 78 copiers in the last four months, respectively. How many copiers will he need to sell this month to maintain an average of at least 77 sales per month ?

Answer 1

GIVEN;

We are told that William sold copiers for the past four months and the figure for each month is given as indicated.

Required;

To determine how many copiers he will need to sell this month to maintain an average of at least 77 sales per month.

Step-by-step solution;

The average sales per month will be calculated using the formula for mean (or average) as shown below;

`Mean=(\Sigma x)/(n)`

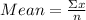
The variables here are;

`\begin{gathered} x=individual\text{ }monthly\text{ }sales \\ \\ n=number\text{ }of\text{ }months \end{gathered}`

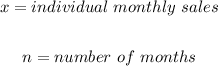
Note at this point that, we have the average already given but we don not have the total sales for all five months. William has sold copiers for the past 4 months and finding the average after this month's sales means we have 5 months' figures. Since we do not have the figure for the current month we shall represent his by letter x.

We can now re-write the formula for the average as follows;

`\begin{gathered} Mean=(\Sigma x)/(n) \\ \\ Mean=(95+41+84+78+x)/(5) \\ \\ Mean=77 \\ \\ Therefore; \\ \\ 77=(95+41+84+78+x)/(5) \end{gathered}`

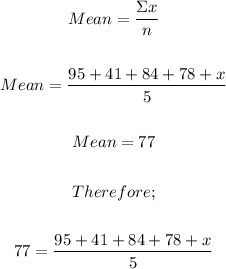
Now we cross multiply;

`\begin{gathered} 77*5=95+41+84+78+x \\ \\ 385=298+x \end{gathered}`

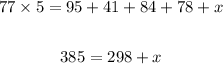
Now we subtract 298 from both sides;

`\begin{gathered} 385-298=298-298+x \\ \\ 87=x \end{gathered}`

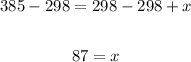
Therefore,

ANSWER:

He will need to sell 87 copiers this month to maintain an average of at least 77 sales per month.