Question

Chords and intersect in circle O as shown in the diagram. Two of the angle measurements are marked. What is mCED?

Answer 1

Most of the information's required to find mCED is already given in the diagram attached with the question. It has a very simple formula.
mCED = (48 + 82)/2
= 130/2
= 65
I hope that this is the answer that you were looking for and it has actually come to your great help.

Answer 2

we know that

The measure of the interior angle is the half sum of the arcs comprising it and its opposite

so

m∠CED=
`(1)/(2) *(arc\ AB+arc\ CD)`

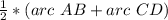
see the attached figure with letters to better understand the problem

`arc\ AB=82`° ------> by central angle

`arc\ CD=48`° ------> by central angle

Substitute the values in the formula above

m∠CED=
`(1)/(2) *(82+48)`

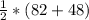
m∠CED=
`(1)/(2) *130`

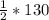
m∠CED=
`65`°

therefore

the answer is

m∠CED=
`65`°