Question

Which is the equation in the slope-intercept form of the line that contains points E & F ?

•4x-y=12
•y-4=4(x-4)
•x-4=4(y-4)
•y=4x-12

Answer 1

y = -4x + 17

Explanation:

Using the slope intercept formula, we can see the slope of line p is ¼. Since line k is perpendicular to line p it must have a slope that is the negative reciprocal. (-4/1) If we set up the formula y=mx+b, using the given point and a slope of (-4), we can solve for our b or y-intercept. In this case it would be 17.

Answer 2

y = 4x - 12

Explanation:

The equation of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

Calculate m using the slope formula

m =
`(y_(2)-y_(1) )/(x_(2)-x_(1) )`

with (x₁, y₁ ) = E(4, 4) and (x₂, y₂ ) = F(2, - 4)

m =
`(-4-4)/(2-4)` =
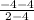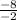`(-8)/(-2)` = 4 , then

y = 4x + c ← is the partial equation

To find c substitute either of the 2 points into the partial equation

Using (4, 4), then

4 = 16 + c ⇒ c = 4 - 16 = - 12

y = 4x - 12 ← equation of line