Answer:
No solutions exist because the situation describes two lines that have the same slope and different y-intercepts.
Explanation:
This question can be solved by a system of equations.
I am going to call the numbers x and y.
A number is equal to twice a smaller number plus 3.
Greater number is x, and smaller is y. So
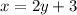
The same number is equal to twice the sum of the smaller number and 1.
This means that

Equaling the values for x:
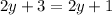
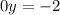
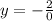
Since division by 0 does not exist, it means that there is no solution for the system.