Question

If a varies directly as b, and a = 28 when b = 7, find b when a = 5.

Answer 1

If a varies directly as b:
a = k b
28 = 7 k
k = 28 : 7 = 4
When a = 5:
5 = 4 b
b = 5 : 4
b = 1.25

Answer 2

Value of b =
`(5)/(4)` =1.25.

Explanation:

Direct Variation states that a relationship between two variables in which one is a constant multiple of the other.

*if one variable changes the other changes in proportion to the first.

*If a is directly proportional to b i.e,

`a \propto b` then it is of the form

a = kb ;where k is constant variation.

Given: a varies directly as b;

then, by definition of direct variation;

we have;

`a = kb` .....[1]

Substitute the value of a =28 and b =7 to solve for k;

`28 = k(7)`

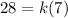
Divide both sides by 7 we have;

`(28)/(7)=(7k)/(7)`

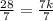
Simplify:

k =4

now, find b using same method when a =5;

then;

after substituting the value of a = 5 and k=4 in [1] ;

`5=(4)b`

Divide by 4 to both sides we get;

`b =(5)/(4)`

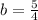
Therefore, the value of b =
`(5)/(4)` =1.25