Question

given the equation 3x − 4y = 8, which equation below would cause a consistent-dependent system? 3x 4y = −8 6x − 8y = 12 9x − 12y = 24 16x 12y = −10

Answer 1

Equation (c) 9x - 12y = 24 would cause a consistent-dependent system

Explanation:

Given equation 3x − 4y = 8

We have to find the equation from the given option that would cause a consistent-dependent system.

A given system of linear equation
`a_1x+b_1y+c=0 \ and\ a_2x+b_2y+c_2=0`

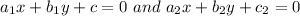
If a consistent system has an infinite number of solutions, it is dependent . When you graph the equations, both equations represent the same line that is slope of both the equation will be same.

that is possible when
`a_2` is multiple of
`a_1` and
`b_2` is multiple of
`b_1` and this multiplication factor is same for both.

Thus, for the given equation 3x − 4y = 8

Only equation (c) 9x - 12y = 24 is a multiple of equation 3x − 4y = 8 with same factor of multiplication 3

Also, the slope of two equation is same
`(3)/(4)`

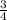
Thus, equation (c) 9x - 12y = 24 would cause a consistent-dependent system

Answer 2

If you would like to know which equation would cause a consistent-dependent system, you can find this using the following steps:

3x - 4y = 8 /*3
3 * 3x - 3 * 4y = 3 * 8
9x - 12y = 24

The correct result would be 9x - 12y = 24.