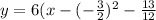Question

What is the first step when rewriting y = 6x2 + 18x + 14 in the form y = a(x – h)2 + k?

Answer 1

The first step in rewriting the equation given to the vertex form is to transpose first the constant term to the other side to simplify the procedure. It is done as follows:

y - 14= 6x² + 18x )

Hope this answer the question.

Answer 2

y = 6(x -(-3/2))^2 - 13/12

Explanation:

Given:
`6x^2 +18x + 14`

We have to write in vertex form y = a(x -h)^2 + k

Step 1: In the given function y = 6x^2 + 18x + 14, the coefficient of x^2 is 6, we need to make it 1, so we take out 6 and factor it.

y = 6(x^2 + 3x + 7/6)

x^2 + 3x + 7/6, the value of b = 3, now divided 3 by 2, then square it.

(3/2)^2 = 9/4

Now add and subtract 9/4

y = 6(x^2 + 3x +9/4 -9/4 + 7/6)

y = 6(x +3/2)^2 -9/4+ 7/6

y = 6(x -(-3/2))^2 +
`((-27 + 14)/(24) )`

Here we simplified -9/4 + 7/6 taking the LCD as 12

`y = 6(x - (-(3)/(2)))^2 +((-13)/(12) )`.

This can be written as

`y = 6(x -(-(3)/(2) )^2 -(13)/(12)`