Question

Points (2, 0) and (0, 3) lie on line k. Which is the slope of the line that is perpendicular to k?

A. 3/2 (A)
B. -3/2 (B)
C. 2/3
D. undefined

Answer 1

Solve is in the picture

Good luck :)

Answer 2

C.
`m=(2)/(3)`

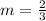
Explanation:

We have been coordinates of two points (2, 0) and (0, 3) that lie on line k. We are asked to find the slope of line that is perpendicular to k.

First of all, we will find the slope of line k using slope formula.

`m=(y_2-y_1)/(x_2-_x1)`, where,

`y_2-y_1`= Difference between two y-coordinates,

`x_2-x_1`= Difference between x-coordinates of same y-coordinates.

Upon substituting our given values in slope formula we will get,

`m=(3-0)/(0-2)`

`m=(3)/(-2)`

`m=-(3)/(2)`

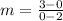
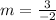
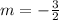
Since the slope of perpendicular to a given line is negative reciprocal of the slope of given line, therefore, the slope of line perpendicular to k will be negative reciprocal of
`m=-(3)/(2)`.

`\text{Slope of line perpendicular to k }=-({-(2)/(3))`

`\text{Slope of line perpendicular to k }=(2)/(3)`

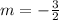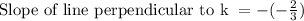
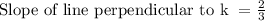
Therefore, option C is the correct choice.