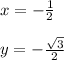Question

The measure of angle θ is 600°. The point (x, y) corresponding to θ on the unit circle is ______.

Answer 1

`x= -(1)/(2)\\\\ y= -(√(3))/(2)`

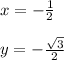
Explanation:

Given : The measure of angle θ is 600°.

To find : The point (x, y) corresponding to θ on the unit circle is ?

Solution :

We know, In the unit circle, the x and y coordinates are the cosine and sine ratios, respectively.

Now, We have given θ = 600°

1 circle= 360°

So, 600° corresponds to 600° - 360° = 240°.

i.e, θ = 360° + 240°

180° < 240° < 270° ⇒ the point is in the third quadrant i.e, x and y coordinates are negative.

Now, The supplementary angle to use notable angles:

240° - 180° = 60°

The sine and cosine of 60° are known:

`\sin 60^\circ = (√(3))/(2)\\\\\cos 60^\circ= (1)/(2)`

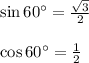
In the unit circle the x-coordinate is the cosine of the angle, and the y-coordinate is the sine of the angle.

Therefore, The coordinates are.

`x= -(1)/(2)\\\\ y= -(√(3))/(2)`

Answer 2

A unit circle has a radius of 1.

Θ = 600°

1 circle = 360°

Θ = 360° + 240°

(x,y) = cos 360° , sin 240°
(x,y) = -0.50 , -0.886

If these were the given choices:
A.) -(3^(1/2))/2 B.)-1(2^(1/2))/2 C.)-1/2 D.) (3^(1/2))/2

x = C) - 1/2
y = A) -(3^1/2) / 2