Question

Choose the equation below whose axis of symmetry is x = 2.

y = x2 + 4x + 2

y = x2 − 4

y = x2 − 2

y = x2 − 4x + 2

Answer 1

Hello,

answer D

A:y=(x+2)²-2 axis: x=-2 No
B:y=x²*4=(x-0)²-4 axis x=0 No
C: y=x²-2=(x-0)²-2 axis x=0 No

D:y=x²-4x+2=x²-4x+4-4+2=(x-2)²-2 axis x=2 Yes.

Answer 2

Option 4 is the correct answer.

Explanation:

There are four equations of a parabola given in the question and we have to find the equation for which axis of symmetry is x = +2

Since line of symmetry of a parabola passes through its vertex so will find - Vertex of all the equations.

(1)
`y=x^(2)+4x-2[tex]</p><p>[tex]y=x^(2)+4x+4-6`

`=(x+2)^(2)-6`

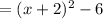
So vertex is ( -2, -6 )

(2)
`y=x^(2)-4=(x-0)^(2) -4`

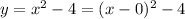
Vertex is ( 0, -4 )

(3)
`y=x^(2) -2`

`y=(x-0)^(2) -2`

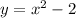
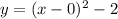
vertex is ( 0, -2 )

(4)
`y=x^(2) -4x+2`

=
`x^(2) -4x+4-2`

=
`(x-2)^(2)-2`

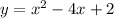
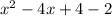
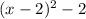
So vertex will be ( +2, -2 )

Out of all equations we find vertex of equation (4) is x=2 and y=-2, so line of symmetry will be x=2.

Option 4 is the correct answer.