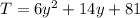Answer:
The required equation is
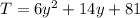
Explanation:
Given : A greenhouse that specializes in growing carnations is divided into sections. The number of plants in each section depends on the number of sprinklers in that section.
Red carnation plants =
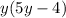
White carnation plants =
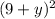
with y sprinklers.
To find : Which simplified expression represents the total number of red and white carnation plants in a section with y sprinklers?
Solution :
Total number = Red carnation + White carnation plants

Now, we solve the equation
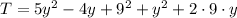
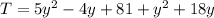
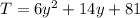
Therefore, The required equation is