Question

Points A and A' have symmetry with respect to the line y = 3. A is point (2, 5). What is point A'?

Answer 1

Answer with explanation:

Coordinates of Point A = (2,5)

It is given that , point A and A' have symmetry with respect to the line y = 3.

→It means that , perpendicular distance of point A from line , y=3 is same as Perpendicular distance of point A' from line , y=3.

→Perpendicular distance of Point A (2,5),from, line ,y=3 is,that is from , point (2,3) is , as x coordinates are same , so

Perpendicular Distance = 5 -3 = 2 unit

X coordinate of Point A'= 2

→Distance from , Point A'(2,k) to line , y=3 that is from , point (2,3) is will be

= 2 unit

→| k -3|=2

k -3 = 2 ∧ 3 -k=2

k=2 +3 ∧ -k=2 -3

k=5 ∧ - k = -1

→k=5 ∧ k=1

So, (2,5) is coordinate of point A and (2,1) is coordinate of point A'.

The Second way is

→Mid point of A(2,5) and A'(x,y) will lie on line, y=3 that is mid point being equal to , (2,3),as drawing perpendicular from point A on line,y=3,gives point (2,3).

`(2 +x)/(2)=2\\\\x+2=4\\\\x=4-2\\\\x=2\\\\ (y+5)/(2)=3\\\\y+5=6\\\\y=6-5\\\\y=1`

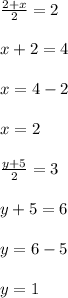
Coordinates of Point A'= (2,1)

Answer 2

To answer this specific question, point A' is (2, 1). I am hoping that this answer has satisfied your query and it will be able to help you in your endeavor, and if you would like, feel free to ask another question.