Question

Solve log4 (y – 9) + log4 3 = log4 81.

Answer 1

The value of y is, 36

Explanation:

Using logarithmic rules:

`\log_b m+ \log_b n = \log_b (mn)`

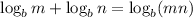
if
`\log_b x = \log_b y` then, x = y

As per the statement:

Given the equation:

`\log_4 (y-9)+\log_4 3 = \log_4 81`

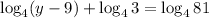
Apply the logarithmic rules;

`\log_4 3(y-9) = \log_4 81`

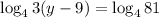
Apply the logarithmic rules; we have;

`3(y-9) = 81`

Divide both sides by 3 we have;

`y-9 = 27`

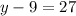
Add 9 to both sides we have;

y = 36

Therefore, the value of y is, 36

Answer 2

Take note that log mn may also be expressed as log m + log n. So, for the given,
log4 (y - 9)(3) = log4 81
Dropping the log4 leaves us with,
(y - 9)(3) = 81
The value of y from the equation is 36.