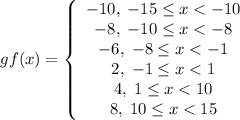Based on the graph, the rule for g(x) should be completed as follows;
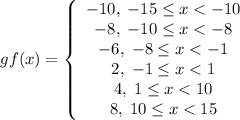
In Mathematics and Euclidean Geometry, a piecewise-defined function is a type of function that is defined by two or more mathematical expressions over a specific domain.
Note: The inequality symbol < or > represents a hollow dot (circle).
The inequality symbol ≤ or ≥ represents a solid dot (circle).
Generally speaking, the domain of any piecewise-defined function is the union of all of its sub-domains. By critically observing the given piecewise-defined function, we have the following domains;
Domain = -15 ≤ x < -10, for g(x) = -10.
Domain = -10 ≤ x < 8, for g(x) = -8.
Domain = -8 ≤ x < -1, for g(x) = -6
Domain = -1 ≤ x < 1, for g(x) = 2
Domain = 1 ≤ x < 10, for g(x) = 4
Domain = 10 ≤ x < 15, for g(x) = 8
In this context, the piecewise function for g(x) can be written as follows;