Question

the graph plots four equations, a, b, c, and d: line a joins ordered pair negative 6, 16 and 9, negative 4. line b joins ordered pair negative 2, 20 and 8, 0. line c joins ordered pair negative 7, negative 6 and 6, 20. line d joins ordered pair 7, 20 and 0, negative 7. which pair of equations has (4, 8) as its solution? equation a and equation c equation b and equation c equation c and equation d equation b and equation d

Answer 1

Answer with explanation:

Eqation of line joining two points (a,b) and (c,d) is given by

`\rightarrow (y-b)/(x-a)=(b-d)/(a-c)`

Eqation of line a, which joins two points (-6,16) and (9,-4) is

`\rightarrow (y-16)/(x+6)=(16-(-4))/(-6-9)\\\\\rightarrow -15 y+240=20 x+120\\\\20x+15y=120\\\\4x+3y=24`

⇒Putting, x=4 and , y=8 in above equation

4 × 4+3×8=16+24=40≠24

Point (4,8) does not lie on this line.

⇒⇒Eqation of line b, which joins two points (2,20) and (8,0) is

`\rightarrow (y-20)/(x-2)=(20-0)/(2-8)\\\\\rightarrow -6 y+120=20 x-40\\\\20x+6y=160\\\\10x+3y=80`

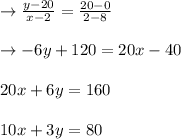
⇒Putting, x=4 and , y=8 in above equation

10 × 4+3×8=40+24=64≠80

Point (4,8) does not lie on this line.

⇒⇒Eqation of line c, which joins two points (7,-6) and (6,20) is

`\rightarrow (y-20)/(x-6)=(20-(-6))/(6-7)\\\\\rightarrow -y+20=26 x-156\\\\26x+6y=176\\\\13x+3y=88`

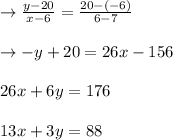
⇒Putting, x=4 and , y=8 in above equation

13× 4+3×8=52+24=76≠88

Point (4,8) does not lie on this line.

⇒⇒Eqation of line d, which joins two points (7,20) and (0,-7) is

`\rightarrow (y-20)/(x-7)=(20-(-7))/(7-0)\\\\\rightarrow 7y-140=27x-189\\\\27x-7y=189-140\\\\27x-7y=49`

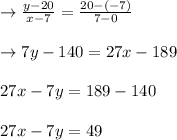
⇒Putting, x=4 and , y=8 in above equation

27× 4-7×8=108-56=52≠24

Point (4,8) does not lie on this line.

⇒None of the two lines has point of Intersection at point (4,8).

Answer 2

The pair of equations that has (4, 8) as its solution are the two equations represented by the lines which intersect at point (4, 8). The lines are line b and line d.
Therefore the equations are equation b and equation a.