Answer: 0.67 grams, because
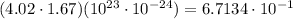
Explanation:
Given : The number of atoms in a closed container =
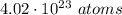
The weight of each atom of the gas =
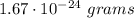
We know that the total mass of atoms of the gas in the container
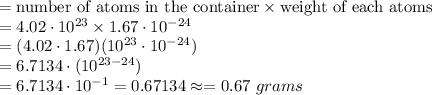
Hence, the approximate total mass of all the atoms of the gas in the container =0.67 grams