Question

What is the surface area of the pyramid to the nearest whole number?

256 cm^2
128 cm^2
64 cm^2
192 cm^2

Answer 1

`A_1=4cm\cdot4cm=16cm^2\\\\A_2=(4cm\cdot6cm)/(2)=(24cm^2)/(2)=12cm^2\\\\The\ surface\ area\ of\ pyramid:\\\\A_S=A_1+4A_2\\\\A_S=16cm^2+4\cdot12cm^2=16cm^2+48cm^2=64cm^2`

Answer 2

64 cm²

Explanation:

To find the area of the square pyramid:

We use the formula;

Area of the square pyramid = B +
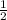`(1)/(2)` p . l

Where b = area of the base

p = perimeter

l = slant height

Area of base = length × breadth

= 4 cm × 4 cm

= 16 cm²

slant height is given as 6 cm

Perimeter = 4 cm + 4 cm + 4 cm + 4 cm = 16 cm

Now we can plug all our variable into the formula;

Area of the square pyramid = B +
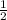`(1)/(2)` p . l

=16 cm² +
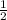`(1)/(2)` × 16 cm × 6 cm

= 16 cm² + 48 cm²

= 64 cm²

( Note; the value of
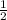`(1)/(2)` × 16 cm × 6 cm = 48 cm²)

Therefore the surface area of the pyramid is 64 cm²