Answer:
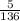
Explanation:
Given: Number of reference books = 2
Number of fiction books =10
Number of non-fiction books =5
Total books=

The probability that she randomly picks up a reference book is given by :-

Without replacement ,the probability that she randomly picks up a non-fiction book is given by :-

Now, the the probability that she randomly picks up a reference book and then, without replacing it, picks up a nonfiction book is given by:-
