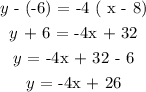Question

Write an equation of the line perpendicular to the given line that contains P. 1 P[8.-6), y = 1/4x +3

Answer 1

Answer:

The equation of the line is y = -4x + 26

Explanations:

The slope-intercept form of the equation of a line is:

y = mx + c

where m = the slope

c = the y-intercept

Comparing the given equation y = 1/4 x + 3 with y = mx + c

m = 1/4

c = 3

The point-slope equation of the line perpendicular to the line y = mx + c is given as:

`y-y_1\text{ = }(-1)/(m)(x-x_1)`

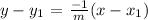
Since the line passes through the point (8, -6)

x₁ = 8, y₁ = -6

-1/m = -1/(1/4) = -4

Substituting the values of x₁, y₁, and -1/m into the equation above:

`\begin{gathered} y\text{ - (-6) = -4 ( x - 8)} \\ y\text{ + 6 = -4x + 32} \\ y\text{ = -4x + 32 - 6} \\ y\text{ = -4x + 26} \end{gathered}`