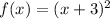Question

Suppose f(x)=x^2.Find the graph of f(x+3)

Answer 1

Replace x with x+3:
f(x+3) = (x+3)²
which is x² translated 3 units left

Answer 2

Answer with explanation:

We are given a parent function f(x) by:

`f(x)=x^2`

i.e. the graph of the function f(x) is a parabola with vertex at (0,0)

Also, we know that the transformation of the type:

f(x) → f(x+k)

is a shift or the translation of the function f(x) k units to the left or to the right depending on k.

if k>0 then the shift is k units to the left.

and if k<0 then the shift is k units to the right.

Here we have to graph the function:

f(x+3) i.e. k=3>0 i.e. the graph of this function is the shift of the graph of the function f(x) 3 units to the left.

i.e. the graph of f(x+3) is a upward open parabola with vertex at (-3,0).

i.e.
`f(x)=(x+3)^2`