Answer
The polynomial identity should be used be Square of Binomial.
To prove
Definition of the Square of binomial
A square binomial is a trinomial that when factored gives you the square of a binomial.
For example, the trinomial x^2 + 2xy + y^2 is a perfect square binomial because it factors to (x + y)^2
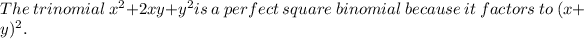
As given
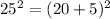
It is written as the trimonial
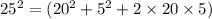
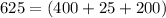
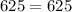
Also
16^2 = (10 + 6)^2
It is written as the trimonial

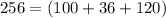
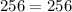
Therefore the Correct answer is Square of Binomial.