Question

How many ways can a dance instructor send 6 of her 9 students to a summer dance program

Answer 1

The question relates to the number of combinations of 9 students taken 6 at a time:

`9C6=(9!)/(6!3!)=(9* 8* 7)/(3*2*1)=84\ ways`

Answer 2

Answer: there are 84 ways to find 6 students from 9 students .

Explanation:

Since we have given that

Total number of students to a summer dance program =9

Total number of students who can be send by dance instructor = 6

Number of ways to choose 6 students from 9 students i.e.

`^9C_6`

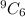
As we know the "Combination formula " i.e.

`^nC_r=(n!)/((n-r)!r!)`

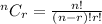
where n denotes the total number of students

r denotes the selected students

So,

`^9C_r=(9!)/((9-6)!6!)\\\\^9C_r=(9!)/(3!* 6!)\\\\^9C_r=(9* 8* 7)/(3* 2* 1)\\\\^9C_r=3* 4* 7\\\\^9C_r=84`

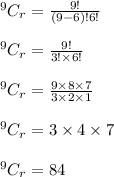
Hence, there are 84 ways to find 6 students from 9 students .