Question

Find a cubic function with the given zeros. Square root of 6, - Square root of 6, -3

Answer 1

`f(x)=(x-\sqrt6)(x+\sqrt6)(x+3)=(x^2-6)(x+3)\\\\=\boxed{x^3+3x^2-6x-18}`

Answer 2

`x^(3) +3x^(2) -6x-18`

Explanation:

We are given zeroes and we need to find a cubic function with these zeroes

zeroes given are :
`√(6) ,  - √(6)  , -3`

Since these are zeroes so these can be written as:

[tex](x-\sqrt{6} )(x-(-\sqrt{6} ))(x-(-3)) = 0[/tex]

⇒
`(x-√(6) )(x+√(6) )(x+3) = 0`

⇒
`(x^(2) +√(6) x- √(6) x -6)(x+3)`

⇒
`(x^(2) -6)(x+3)`

⇒
`x^(3) +3x^(2) -6x-18`

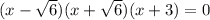
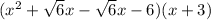
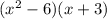
Thus the required cubic function is
`x^(3) +3x^(2) -6x-18`