Question

Which of the following circles have their centers on the x-axis? Check all that apply.

A. (x - 2)2 + (y + 3)2 = 2
B. (x - 0)2 + (y - 3)2 = 23
C. (x - 0)2 + (y - 0)2 = 72
D. (x - 4)2 + (y - 0)2 = 74

Answer 1

c and d

Explanation:

Answer 2

`Circles\thinspace (x - 0)^2 + (y - 0)^2 = 72\thinspace and \thinspace (x - 4)^2 + (y - 0)^2 = 74\text{ have their centers on the x-axis.}`

Explanation:

Given the equations of circle. we have to find the equation of circle whose center on the x-axis.

The standard form of equation of circle is

`(x-h)^2+(y-k)^2=r^2`

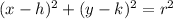
where (h,k) is center and r is radius.

Given equations are

`(x - 2)^2 + (y + 3)^2 = 2` Center:(2,-3)

`(x - 0)^2 + (y - 3)^2 = 23` Center:(0,3)

`(x - 0)^2 + (y - 0)^2 = 72` Center:(0,0)

`(x - 4)^2 + (y - 0)^2 = 74` Center:(4,0)

The points with ordinate 0 lies on x-axis

Hence,
`Circles\thinspace (x - 0)^2 + (y - 0)^2 = 72\thinspace and \thinspace (x - 4)^2 + (y - 0)^2 = 74\text{ have their centers on the x-axis.}`