Question

what is the inverse function of h(x)= -3/5x 1/2? h-1(x)=-5x 5 h-1(x)=-5/3x 5/6 h-1(x)=5/3x - 5/6 h-1(x)=5x-5

Answer 1

Hello,

Answer B

h(x)=-3/5 x+1/2 ==>x=-3/5y+1/2
==>3/5 y=-x+1/2
==>y=-3/5 x+(1/2)/(3/5)
==>y=-3/5 x+5/6

Answer 2

B.
`h^(-1)(x)=(-5x)/(3)+(5)/(6)`

Explanation:

We have been given a function
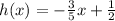`h(x)=-(3)/(5)x+(1)/(2)`. We are asked to find the inverse function of our given function.

First of all, we replace h(x) by y as:

`y=-(3)/(5)x+(1)/(2)`

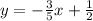
To find inverse of our given function we will interchange x and y variables and solve for y.

`x=-(3)/(5)y+(1)/(2)`

Upon subtracting 1/2 from both sides of equation, we will get:

`x-(1)/(2)=-(3)/(5)y+(1)/(2)-(1)/(2)`

`x-(1)/(2)=-(3)/(5)y`

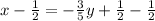
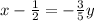
Let us have a common denominator.

`(2x)/(2)-(1)/(2)=-(3)/(5)y`

`(2x-1)/(2)=-(3)/(5)y`

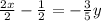
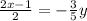
Multiplying both sides by
`-(5)/(3)`,

`-(5)/(3)*(2x-1)/(2)=-(5)/(3)*-(3)/(5)y`

`(-10x+5)/(2*3)=y`

`(-10x)/(6)+(5)/(6)=y`

`(-5x)/(3)+(5)/(6)=y`

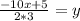
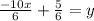
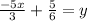
Replacing y by
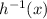`h^(-1)(x)` we will get,

`h^(-1)(x)=(-5x)/(3)+(5)/(6)`

Therefore, the option B is the correct choice.

Therefore,