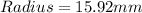Question

A circle has a central angle of 90 degrees . The arc length is 25 mm . What is the radius of the circle ?

Answer 1

Step-by-step explanation:

Give;

We are told of a circle with a central angle measure of 90 degrees. Also the length of the arc formed by the radii is 25 mm.

Required;

We are required to calculate the radius of the circle.

Step-by-step solution;

To solve this problem, we first take note of the formula for calculating the length of an arc, and this is;

`\begin{gathered} LENGTH\text{ }OF\text{ }AN\text{ }ARC: \\ \\ LOA=(\theta)/(360)*2\pi r \end{gathered}`

The variables here are;

`LOA=25,\theta=90\degree,\pi=3.14,r=?`

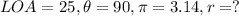
We can now substitute the known values and we'll have;

`\begin{gathered} 25=(90)/(360)*2*3.14* r \\ \\ 25=(1*2*3.14* r)/(4) \end{gathered}`

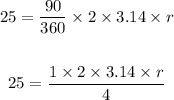
Now we can cross multiply;

`\begin{gathered} (25*4)/(2*3.14)=r \\ \\ 15.923566879=r \end{gathered}`

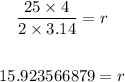
We can round this to the nearest hundredth and we now have;

ANSWER:

`Radius=15.92mm`