Question

which statement describes the graph of f(x) = 4x2 20x 25? the graph does not intersect the x-axis. the graph touches the x-axis at (–2.5, 0). the graph intersects the x-axis at (–0.4, 0) and (0.4, 0). the graph intersects the x-axis at (2, 0) and (5, 0).

Answer 1

The graph touches the axis at (-2.5,0).

Answer 2

The graph of the quadratic function
`y=ax^2+bx+c:`

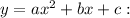
• does not intersect the x-axis, when
  `D=b^2-4ac<0;`
• touches the x-axis at one point, when
  `D=b^2-4ac=0;`
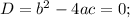
• intersects x-axis at two points, when
  `D=b^2-4ac>0.`

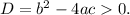
For the function
`y=4x^2+20x+25,` the discriminant D is

`D=20^2-4\cdot 4\cdot 25=400-400=0.`

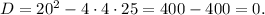
This means that the graph of the function touches the x-axis at one point.

Since
`y=4x^2+20x+25=(2x+5)^2,` then the tangent point has x-coordinate
`x=-2.5` (value at which y=0).

Answer: correct choice is B