Question

Determine whether f(x) and g(x) are inverses. Show your work.

Answer 1

Answer:

f(x) and g(x) are inverses

Step-by-step explanation:
`\begin{gathered} f(x)\text{ = }(1)/(x-5)+8 \\ g(x)\text{ = }\frac{1}{x\text{ - 8 }}\text{ + 5} \end{gathered}`

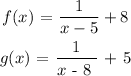
To find:

To determine whether f(x) and g(x) are inverses

For two function to be inverse of ech other, f(g(x))) = x and g(f(x)) = x. We will find the value/expression of f((g(x)) and g(f(x))

`\begin{gathered} f(g(x))\text{ = }\frac{1}{\frac{1}{x\text{ - 8}}+\text{ 5 -5}}+8 \\ \\ f(g(x))\text{ = }\frac{1}{\frac{1}{x\text{ - 8}}}\text{ + 8 = 1 }*(x-8)/(1)\text{ + 8} \\ \\ f(g(x))\text{ = x - 8 + 8} \\ f(g(x))\text{ = x} \end{gathered}`
`\begin{gathered} g(f(x))\text{ = }\frac{1}{\frac{1}{x\text{ - 5}}+8-8}\text{+ 5} \\ g(f(x))\text{ = }(1)/((1)/(x-5))+5 \\ g(f(x))\text{ = x - 5 + 5} \\ g(f(x\text{\rparen\rparen = x} \end{gathered}`

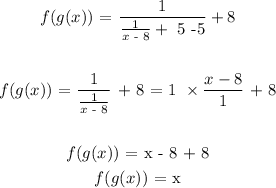
Since f(g(x)) = x and g(f(x)) = x

Then f(x) and g(x) are inverses