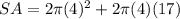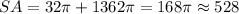Question

Which cylinders have a surface area of approximately 528 square units?

Answer 1

Step-by-step explanation

Background

• Diameter: ,line that touches two opposite points in a circumference and passes through the center of the circle.

,

• Radius: ,the distance from the center to any point in the circumference. Also, it is half the diameter.

The surface area (SA) of a cylinder is given by:

`SA=2\pi r^2+2\pi rh`

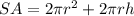
where r represents the radius and h represents the height.

Based on the given figures, we can conclude that:

• The cylinder in the upper left corner has a diameter of 12 and a height of 8, meaning r = 12/2 = 6 and h = 8.

• The cylinder in the upper right corner has a radius of 2 and a height of 12, meaning r = 2 and h = 12.

• The cylinder in the lower right corner has a radius of 7 and a height of 5, meaning r = 7 and h = 5.

• The cylinder in the lower left corner has a diameter of 8 and a height of 17, meaning r = 8/2 = 4 and h = 17.

Now, we can replace these values in the formula:

• Cylinder in the upper left corner

`SA=2\pi(6)^2+2\pi(6)(8)`
`SA=2\pi(36)+2\pi(48)`
`SA=72\pi+96\pi`
`SA=168\pi\approx527.78\approx528`

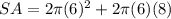
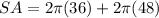
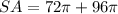
• Cylinder in the upper right corner

`SA=2\pi(2)^2+2\pi(2)(12)`
`SA=8\pi+48\pi=56\pi`
`SA=56\pi\approx175.93\approx176`

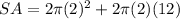
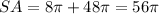
• Cylinder in the lower right corner

`SA=2\pi(7)^2+2\pi(7)(5)`
`SA=98\pi+70\pi=168\pi\approx528`

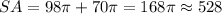
• Cylinder in the lower left corner

`SA=2\pi(4)^2+2\pi(4)(17)`
`SA=32\pi+1362\pi=168\pi\approx528`