Question

The graph of f(x) = |x| is transformed to g(x) = |x + 1| – 7. On which interval is the function decreasing?

Answer 1

(–∞, –1) is the answer

Explanation:

Answer 2

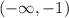`(-\infty,-1)` interval is the function decreasing.

Explanation:

Given : The graph of
`f(x) = |x|` is transformed to
`g(x) = |x+1|-7`

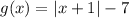
To find : On which interval is the function decreasing?

Solution :

First we plot the graph of both the functions,

The graph of
`f(x) = |x|` is shown with black line.

The graph of
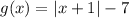`g(x) = |x+1|-7` is shown with violet line.

Refer the attached figure below.

The graph shows the interval over which it is increasing or decreasing.

As we notice it is increasing on the interval
`(-1,\infty)`

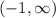
Decreasing on
`(-\infty,-1)`

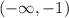
Therefore,
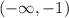`(-\infty,-1)` interval is the function decreasing.