Answer:
The least minimum value is attained by:
First function---- f(x)
Coordinate of the point: (-1,-3)
Explanation:
We are given a equation of first function as:
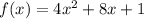
The graph of this function is a parabola which is open upward and the vertex of the parabola is at (-1,-3)
Since, the parabola is open upward hence the vertex of the parabola is the point of minima of the parabola.
The minimum value of the function f(x) is:
-3
Now we are given a table of values of the second function i.e. g(x) as:
x g(x)
−2 2
−1 0
0 2
1 8
Clearly by looking at the values we see that:
The minimum value attained by the function g(x) is:
0
The least minimum value is attained by:
First function--- f(x)
The coordinates of the minimum point are: (-1,-3)