Question

The height of an isosceles triangle is 12cm. The base of the triangle is 18cm. Work out the length of the equal sides

Answer 1

The length of the equal sides is 15 centimeters.

Explanation:

Since, base and height of the triangle are perpendicular to each other. In an isosceles triangle, the value of each equal length (
`l`), measured in centimeters, can be determined by the following Pythagorean identity:

`l = \sqrt{(b^(2))/(4)+h^(2)}` (1)

Where:

`b` - Base of the triangle, measured in centimeters.

`h` - Height of the triangle, measured in centimeters.

If we know that
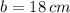`b = 18\,cm` and
`h = 12\,cm`, then the value of each equal length within the isosceles triangle is:

`l =\sqrt{((18\,cm)^(2))/(4)+(12\,cm)^(2)}`

`l = 15\,cm`

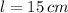
The length of the equal sides is 15 centimeters.