Question

How do you determine resultant force in physics?

Answer 1

You have to know the magnitude and direction of the individual forces. Let's say you have 3 forces acting on a body.
F1 = 45 N at 45°F2 = 60 N at 120°F3 = 75 N at 270°
Now you have to resolve the forces into their horizontal (x) and vertical (y) components.
F1 = <45 cos 45°, 45 sin 45°> N = <31.8, 31.8> NF2 = <60 cos 120°, 60 sin 120°> N = <-30, 52> NF3 = <75 cos 270°, 75 sin 270°> N = <0, -75> N
Add the horizontal component (1st number) of each force to find the horizontal component of the resultant force (Fx).
Fx = 31.8 N + (-30 N) + 0 N = 1.8 N
Do the same with the vertical components to find Fy.
Fy = 31.8 N + 52 N + (-75 N) = 8.8 N
Now find the magnitude of the resultant force

`|F| = √(F_x^2 + F_y^2)=√(1.8^2 + 8.8^2) = 8.98 N`

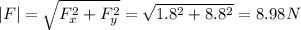
Now find the direction of the resultant force.

`\theta=\tan^(-1)(F_y)/(F_x)=tan(-1)(8.8)/(1.8)=78.4^o`

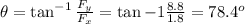
The next thing you should do is figure out which quadrant the resultant force is in. The arctan function only has a range from -90° to 90°, so if the force is outside the first quadrant you'll have to use your knowledge of reference angles to find the corresponding angle between 0° and 360°

We have a positive Fx and a positive Fy, so F is in the first quadrant, and 78.4° is the correct angle.

The resultant force is 8.98 N at 78.4°.