Question

William opened two investment accounts The first year, these investments, which totaled $2600 yielded $137 in simple interest Part of the money was invested at 5.5% and the rest at 5%. How much was invested at each rate?

Answer 1

• The amount invested at 5.5% = $1400

,

• The amount invested at 5% = $1200

Explanation:

The total investment = $2,600

• Let the amount invested at 5.5% = x

,

• Let the amount invested at 5% = y

`x+y=2600\cdots(1)`

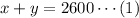
The total yield on the investments = $137.

`\begin{gathered} \text{ Interest on \$x}=0.055x \\ \text{ Interest on \$y}=0.05y \\ \implies0.055x+0.05y=137\cdots(2) \end{gathered}`

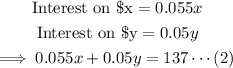
Thus, we have a system of linear equations:

`\begin{gathered} x+y=2600 \\ 0.055x+0.05y=137 \end{gathered}`

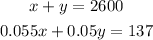
From equation (1), x=2600-y. Substitute this into equation (2):

`\begin{gathered} 0.055(2600-y)+0.05y=137 \\ 143-0.055y+0.05y=137 \\ -0.005y=137-143=-6 \\ y=(-6)/(-0.005) \\ y=1200 \end{gathered}`

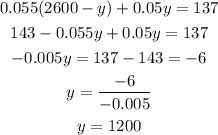
Finally, we find the value of x:

`x=2600-y=2600-1200=1400`

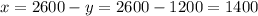
Therefore:

• The amount invested at 5.5% = $1400

,

• The amount invested at 5% = $1200