Question

Identify the constant of proportionality for each graph and write equations

Answer 1

k=2, y=2x

K=7, y=7x

k=1/3, y=x/3

Step-by-step explanation

When two variables are directly or indirectly proportional to each other, then their relationship can be described as y = kx

so, to find the constant we need

`\begin{gathered} y=kx \\ \text{divide both sides by x} \\ (y)/(x)=(kx)/(x) \\ (y)/(x)=k \end{gathered}`

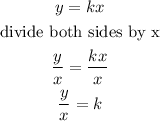
therefore, to find k just take a point from the line and divide y by x,

hence

Step 1

graph 6

take a point

P=(2,4)

now, replace to find k

`\begin{gathered} k=(4)/(2) \\ k=2 \end{gathered}`

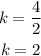
therefore, for 6 the constant is 2

and the equation is

`y=2x`

Step 2

graph 7

point=(3,21)

replace

`\begin{gathered} k=(y)/(x) \\ k=(21)/(3) \\ k=7 \end{gathered}`

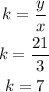
therefore, for 7 the constant is 7

`\begin{gathered} \text{and the equation would be } \\ y=7x \end{gathered}`

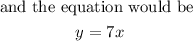
Step 3

Finally, graph 8

point =(9,3)

replace

`\begin{gathered} k=(3)/(9) \\ k=(1)/(3) \end{gathered}`

therefore, for 8 the constant is 1/3

and the equation would be

`y=(x)/(3)`

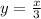
I hope this helps you