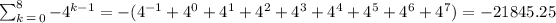Question

Evaluate the geometric series described below.a1 = -1, r = 4, n = 8

Answer 1

First let's see the expression for a geometric series:

`\sum ^n_(k\mathop=0)a\cdot r^k`

In this problem r=4 and n=8. a is not given, however, you do have the second term of the series:

`a_1=-1`

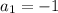
This means that, when k=1, the term equals 1:

`a\cdot4^1=-1`

So, we can figure out the value of a from here:

`a=-(1)/(4)`

So, the complete geometric series given is:

`\sum ^8_(k\mathop=0)(-1)/(4)\cdot4^k=\sum ^8_(k\mathop=0)-4^(k-1)`

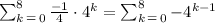
And finally you just have to evaluate the sum:

`\sum ^8_(k\mathop=0)-4^(k-1)=-(4^(-1)+4^0+4^1+4^2+4^3+4^4+4^5+4^6+4^7)=-21845.25^{}^{}^{}^{}^{}`