Question

What is the value of the fourth term in geometric sequence for which a1 = 15 and r = 1/3?

Answer 1

so you express fraction.
15 x (1/3)^ 3=15/27=5/9

Answer 2

the fourth term is 5/9

Explanation:

a sequence is defined like this:

1, 2, 3,4…….. n

1= first term

2=second term

3= third term

n=term n of the secuence

a geometric sequence is defined as follows

`a,ar,ar^(2) ,ar^(3) ,..............ar^(n-1)`

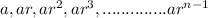
The General formula to calculate the term n of a geometric sequence is:

`term(n)=ar^(n-1)` Formula (1)

We can calculate any term of the sequence by replacing the information known in the formula (1):

For this case: a=a1=15 , r=1/3

`term(n)=15*(1/3)^(n-1)` Formula (2)

We replace n=4 in the formula 2

`term(4)=15*(1/3)^(4-1) \\`

`term(4)=15*(1/3)^(3)`

`term(4)=15*(1/27)`

`term(4)=5/9`

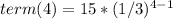
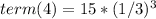
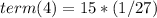
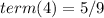
The fourth term of the geometric sequence is 5/9