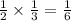Answer: a)
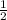
b)
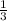
c)
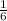
Explanation:
The probability of a question from the material that was read = 60 % = 0.6
The probability of a question from the material that was not read = 40 % = 0.4
The probability of a right answer out of 5 questions
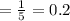
While, the probability of wrong answer = 1 - 0.2 = 0.8
a) Thus, by the definition of conditional probability,
The probability of getting a right answer when it is given that it is from the unread material
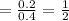
b) The probability of getting a right answer when it is given that it is from the read material
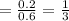
c) And, overall probability of a correct answer
= The correct answer is from the read material or the correct answer is from the unread material
=